JKBOSE 9th Class Science Solutions Chapter 3 Work, Energy and Power
JKBOSE 9th Class Science Solutions Chapter 3 Work, Energy and Power
JKBOSE 9th Class Science Solutions Chapter 3 Work, Energy and Power
Jammu & Kashmir State Board JKBOSE 9th Class Science Solutions
J&K class 9th Science Work, Energy and Power Textbook Questions and Answers
BASIS AND BASICS
◆ All living beings need food.
◆ In order to survive all living beings have to do some basic activities for which energy is required. This energy they get from food they eat.
◆ Machines too need fuels like petrol and diesel for energy.
◆ In our daily life we consider any useful physical or mental activity as work. From science point of view for doing work two conditions must be satisfied : (1) some force must act on the object (ii) the object must be displaced in the direction of force.
◆ Work done by the force acting on a body is equal to the product of magnitude of the force the force and the displacement in the direction of force.
◆ When force acts in a direction opposite to the direction of displacement produced, then the work done is negative.
◆ When force acts in the direction of displacement then the work done is positive.
◆ For us the sun is the major natural source of energy. In addition to this, we can also get energy from nuclei of atoms, from interior of earth and tides.
◆ If a body has capacity to do work then it is said to possess energy.
◆ The object which does work loses energy and the object on which work is done gains energy.
◆ Energy is present in different forms as for example kinetic energy, potential energy, heat energy, chemical energy, electric energy and light energy.
◆ The sum total of potential and kinetic energy of the body is called mechanical energy.
◆ The energy present in the body due to its motion is called its kinetic energy.
◆ The kinetic energy of an object increases with the increase of its velocity.
◆ When an object is raised to some height above the ground, the work done against gravitational force is stored in the object as potential energy.
◆ We can transform one form of energy to another form.
◆ According to law of conservation of energy, energy can neither be created nor destroyed but it can only be transformed from one form to another form total energy before and after transformation always remains constant.
◆ The rate of doing work or transformation of energy is called power.
◆ The unit of power is watt
◆ 1 kilowatt = 1000 watt
IMPORTANT TERMS/FACTS TO MEMORISE
⇒ Energy. The capacity of doing work is called energy.
⇒ Kinetic Energy. The capacity of the bodies to do work due to motion present in them is called kinetic energy.
⇒ Potential Energy. The capacity of an object to do work due to change in its position or configuration is called its potential energy.
⇒ Law of Conservation of Energy. Energy can neither be created nor destroyed but it can be changed from one form into another form.
⇒ Joule. If one Newton (N) of force acts on a body of 1 kg and displaces it through 1 m than work done on the body is one joule.
⇒ Power. The rate at which energy is supplied or consumed is called power. The unit of power is watt (W).
Power = Energy supplied / Time
⇒ Watt. If a source supplies or consumes energy at the rate of 1 Joule (J) per second then the power of the source is said to be one watt.
⇒ Work. If force acting on a body displaces the body in the direction of force then work is said to be done by the force.
Work = Force × Displacement
i.e. W = F × S
TEXTBOOK QUESTIONS (SOLVED)
Q. 1. A force of 7 N acts on an object. The displacement is, say 8 m in the direction of the force. Let us take it that the force acts on the object through the displacement. What is the work done in this case ?

Sol.— Here force (F) = 7 N
Displacement (S) = 8 m
Work done (W) = ?
We know, W = F × S
= 7 N × 8 m
= 56 N – m
= 56 J Ans.
Q. 2. When do we say that work is done ?
Ans.— Work. When a force is applied on a body and the body gets displaced in the direction of force, then work is said to be done.
Work (W) = Force (F) × Displacement (S)
Q. 3. Write an expression for the work done when one when a force is acting on an object in a force the direction of its displacement.
Ans.— When the displacement of the object is in the direction of force then,
Work done (W) = Force (F) x Displacement (S)
Q. 4. Define 1 J of work.
Ans.— Joule. Work done on the body is said to be 1 J if 1N of the force acting on it displaces the body in its own direction through a distance of 1 m.
We know, W = FXS
or, 1 J = 1 N × 1 m
Q. 5. A pair of bullocks exerts a force of 140 N on a plough. The field being ploughed 15 m long. How much work is done in ploughing the length of the field ?
Sol.— Force exerted by bullocks (F) = 140 N
Length of the field ploughed (S) = 15 M
Work done in ploughing the field (W) = ?
We know, W = F × S
W = 140 N × 15 m
= 2100 N – m
= 2100 J Ans.
Q. 6. What is kinetic energy of an object ?
Ans.— Kinetic Energy. Kinetic energy of an object is the quantity of motion possessed by it.
Kinetic energy of an object of mass ‘m’ moving with velocity ‘v’ is = 1/2 mv ²
S.I. unit of kinetic energy is Joule
Examples. (i) Stone in motion
(ii) Blowing wind
(iii) Rotating wheel
Q. 7. Write an expression for the kinetic energy of an object.
Ans.— Kinetic energy of an object of mass ‘m’ moving with velocity ‘v’ is
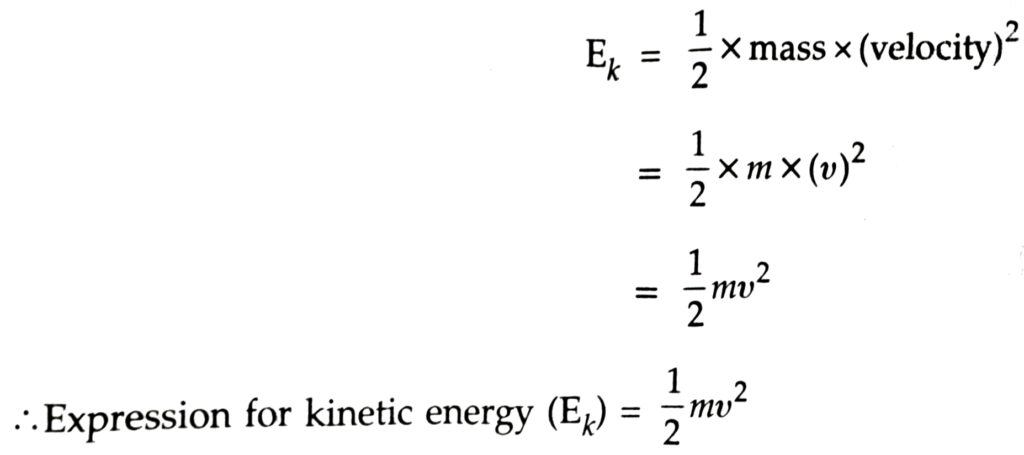
Q. 8. Define Average Power.
Ans.— Average Power. It is defined as the ratio of total energy consumed to the total time taken.∴
∴ Average Power (Pav) = Total energy consumed / Total time taken
TEXTBOOK EXERCISES (SOLVED)
Q. 1. Look at the activities listed below. Reason out whether or not work is done in the light of your understanding of the term ‘work’.
(i) Suma is swimming in a pond.
(ii) A donkey is carrying a load on its back.
(iii) A wind-mill is lifting water from a well.
(iv) A green plant is carrying out photosynthesis.
(v) An engine is pulling a train.
(vi) Food grains are getting dried in the sun.
(vii) A sail boat is moving due to wind energy.
Ans.— (i) Suma while swimming is applying her muscular force in a particular direction and gets displaced. Therefore, work is being done by Suma.
(ii) The load being carried by donkey is acting in the downward direction perpendicular to the horizontal direction of displacement. And when the force acts perpendicular to the direction of displacement then no work is done.
Therefore, donkey is not doing any work.
(iii) Work is being done because in lifting water, the displacement as well as force are in vertically upward direction.
(iv) A green plant carrying photosynthesis does no work since neither there is force applied nor any displacement in direction of force applied.
(v) An engine pulling a train is doing work since displacement is in direction of force applied.
(vi) No work is done on food grain. However part of heat supplied coverts moisture of grains into steam which rises up increasing P.E.
(vii) Work is being done since force and displacement is there in the same direction.
Q. 2. An object thrown at a certain angle to the ground moves in a curved path and falls back to the ground. The initial and final points of the path of object lie on the same horizontal line. What is the work done by the force of gravity on the object ?
Ans.—The work done by the force of gravity will be zero. This is because the displacement is in a horizontal direction while the force is acting vertically downward perpendicular to this direction of displacement.

In this situation θ = 90°
∴ cos θ = cos 90° = 0
Now work done (W) = F cos θ × S
= F × 0 × S
W = 0
Q. 3. A battery lights a bulb. Describe the energy changes ivolved in the process.
Ans.— Chemical energy of the chemicals in the battery is first being converted to electric energy. Then the electric energy of the battery is converted into heat energy and light energy by the bulb.
Q. 4. Certain force acting on a 20 kg mass changes its velocity from 5 m s -1 to 2 m s -¹. Calculate the work done by the force.
Sol.— Here mass (m) = 20 kg
Initial velocity (u) = 5 m s-1
Final velocity (v) = 2 m s-1
Initial kinetic energy of the body (Ek1) = 1/2 mu²
= 1/2 × 20 × (5)²
= 1/2 × 20 × 5 × 5
= 250 J
Final kinetic energy of the body (Ek2) = 1/2 mv²
= 1/2 × 20 × (2)²
= 1/2 × 20 × 2 × 2
= 40 J
∴ Work done by the force = Change in kinetic energy
= Final kinetic Energy – Initial kinetic energy
= 40 J – 250 J
Negative sign shows that there is decrease in velocity due to opposing force which is doing work.
Q. 5. A mass of 10 kg is at a point A on a table. It is moved to a point B. If line joining A and B is horizontal, what is the work done on the object by gravitational force ? Explain your answer.
Solution.— An object of mass 10 kg is displaced in the horizontal direction from point A to point B but the gravitational force is acting vertically downward which makes an angle of 90° with the direction of displacement.
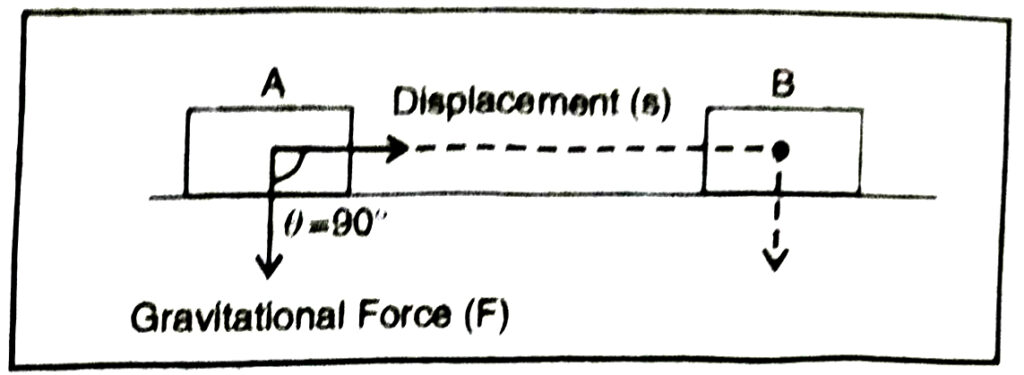
∴ Work done by the gravitational force (W) = F cos θ × S
= F cos 90° × S
= F × 0 × S
= 0 Ans.
Q. 6. The potential energy of a freely falling object decreases progressively. Does this violate the law of conservation of energy ? Why ?
Ans.— It does not violate the law of conservation of energy. When the height of freely falling body decreases, its potential energy decreases but kinetic energy increases. Kinetic energy increases by the same amount as potential energy has decreased. At any time the sum of kinetic energy and potential energy remains conserved.
Q. 7. What are the various energy transformations that occur when you are riding a bicycle ?
Ans.— When we are riding a bicycle and pedalling it, the energy of our muscles gets transformed into heat energy and kinetic energy. This kinetic energy is used in doing work against the frictional energy offered by the road.
Q. 8. Does the transfer of energy takes place when you push a huge rock with all your might and fail to move it ? Where is the energy you spent going ?
Ans.— Although you have not been able to move the heavy rock, you are very much tired and this has reduced your energy. Since we have failed to move the heavy rock, work appears to be zero.
While pushing the stone, you had to stretch your muscles, heart had to pump more blood and in making these changes, your energy is definitely lost. The work done by you on your body is not zero. You may have to eat some food to compensate for the work done by your muscles and heart.
Q. 9. A certain household has consumed 250 unit of electric energy during a month. How much energy is this in joules ?
Sol.— We know, 1 unit of energy = 1 kilowatt hour (1kWh)
= 1 kW x 1h
= (1 x 1000 watt) × (1 x 60 × 60 s)
= 36 × 10⁵ J
= 3.6 × 10⁶ J
∴ 250 units of energy = 250 × 3.6 x 10⁶ J
= 900 × 10⁶ J
= 9 × 10⁸ J Ans.
Q. 10. An object of mass 40 kg is raised to a height of 5 m above the ground. What is its potential energy? If the object is allowed to fall, find its kinetic energy when it is half-way down. Take g = 10 m s-2.
Sol.— Here mass of the object (m) = 40 kg
Height above the ground (h) = 5 m
Acceleration due to gravity (g) = 10 m s-2
Potential energy of the object at a height of 5 m (Ep) = m × g × h
= 40 × 10 × 5 J
= 2000 J Ans.
Let v be the velocity of the object when it has come halfway down
Distance moved by the object (S) = 5/2 = 2.5 m
Using, v2– u2 = 2gS
v2 – (0)2 = 2 × 10 × 2.5
v2 = 2 × 25
or, v2 = 50
Kinetic energy of the object on reaching half way down (Ek) = 1/2 mv²
= 1/2 × 40 × 50
= 1000 J Ans.
Q. 11. What is the work done by the force of gravity on a satellite moving round the earth ? Justify your answer.
Ans.— When a satellite moves around the earth the force of gravity is directed inward along the radius of the circular path while the direction of motion is along the tangent which is perpendicular to the radius. In this way force of gravity and displacement are mutually at right angle en to each other as a result of which the work done on the satellite is zero.
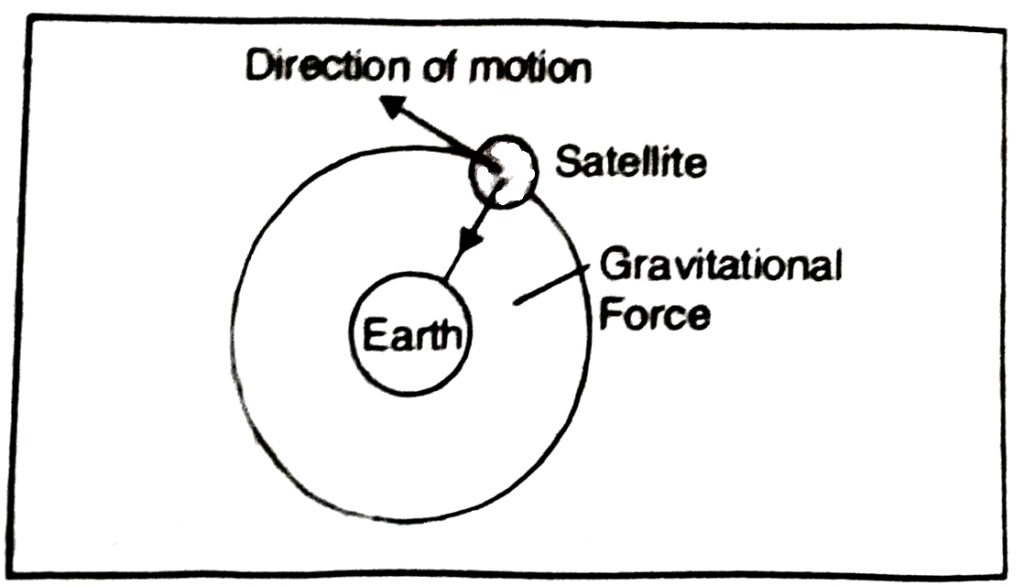
We know, work done (W) = F cos θ × S
= F cos 90° × S
= F × 0 × S
= 0
Q. 12. Can there be a displacement of any object in the absence of any external force ?
Ans.— In the absence of any external force the displacement of the object is possible if the object is moving with a uniform velocity. And if the object is in the state of rest then the displacement is not possible in the absence of external force.
Q. 13. A person holds a bundle of hay over his head for 30 minutes and gets tired. Has he done some work or not ? Justify your answer.
Ans.— A person holds a bundle of hay over his head for 30 minutes and got tired but the force of gravity acting on the bundle did not displace the bundle of hay in the direction of force of gravity. Since there is no displacement in the direction of force, therefore, no work is said to be done by him.
Q. 14. An electric heater is rated 1500 W. How much energy does it use in 10 hours ?
Sol.— Given, Power of the heater (P) = 1500 W
Time for which heater is used (t) = 10 hr
Energy used by the heater in 10 hours (E) = ?
We know, energy used = Power × Time
= 1500 Watt × 10 hrs
= 15000 Wh
= 15000 / 1000 kWh
= 15 kWh Ans.
= 15 units Ans.
Q. 15. Illustrate the law of conservation of energy by discussing the energy changes which occur when we draw a pendulum bob to one side and allow it to oscillate. Why does the bob eventually come to rest ? What happens to its energy eventually ? Is it a violation of the law of conservation of energy ?
Ans.— Energy transformation in oscilated pendulum :
Initially bob is at rest at its mean position, thus in kinetic energy is zero. We can consider its potential energy equal to zero in this position.
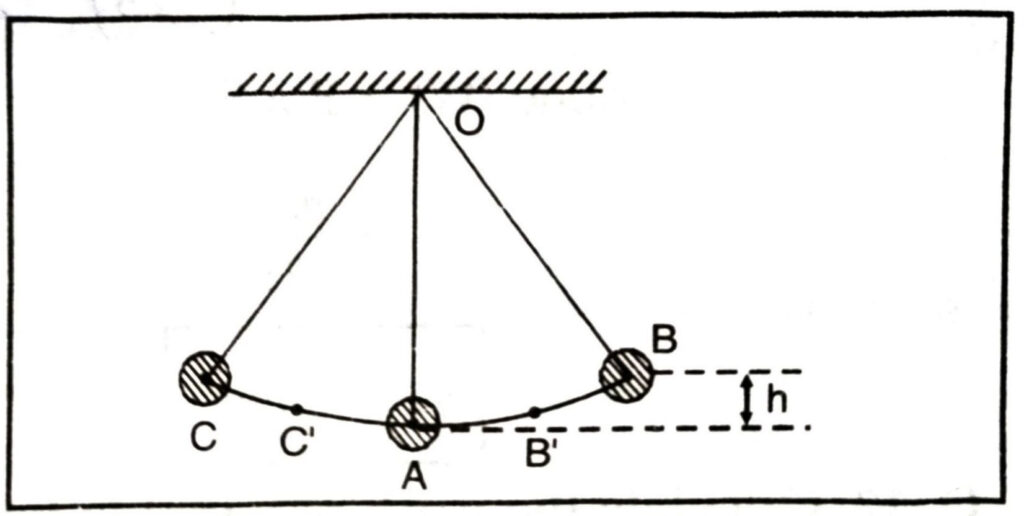
When we take the bob of the pendulum to one side its height goes on increasing and we have to do some work against the force of gravity. This work is stored in the bob as its potential energy. Thus, when a bob is released from one of its extreme position, i.e. state of maximum displacement B at this position its kinetic energy is zero and potential energy is maximum. Now, the bob is in motion and is moving towards its mean position A its height goes on decreasing that means its potential energy also goes on decreasing whereas its velocity goes on increasing and hence, its kinetic energy increases. Because bob is moving through the air, thus, some energy is consumed against the force of friction due to air. This causes increase in speed of molecules of air and thus, kinetic energy of the molecules increase. At the mean position, kinetic energy of the bob becomes maximum and potential energy becomes minimum. Due to inertia of motion bob does not stop here but it moves to the other side of its mean position.
Its height again starts increasing so that potential energy also increases, but kinetic energy continues to decrease. When bob reaches at the extreme position ‘O’ its potential energy becomes maximum and kinetic energy becomes zero. Bob does not stop here it tev comes back towards its mean position ‘A’. At every point of its motio point of its motion, sum of kinetic energy and potential energy of the bob along with energy of air molecules remains constant. Thus, during oscillation of the bob of a simple pendulum total energy remains conserved.
Amplitude of the simple pendulum depends on the total energy of the bob. The energy transfered to the molecules of the air by the oscillating bob can never be recovered. Thus total energy of the bob goes on decreasing. When, the bob transfers whole of its energy to the molecules of the air then its total energy becomes zero and it comes to rest at its mean position.
Thus there is no violation of the law of conservation of energy.
Q. 16. An object of mass ‘m’ is moving with velocity ‘v’ . How much work should be done on the object in order to bring the object at rest ?
Sol.— Let an object of mass m be initially moving with velocity v and finally be brought to rest by the application of an opposing force F after covering a distance S.
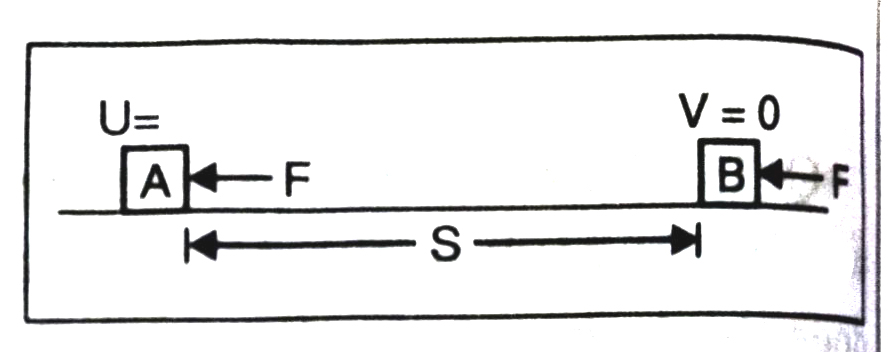
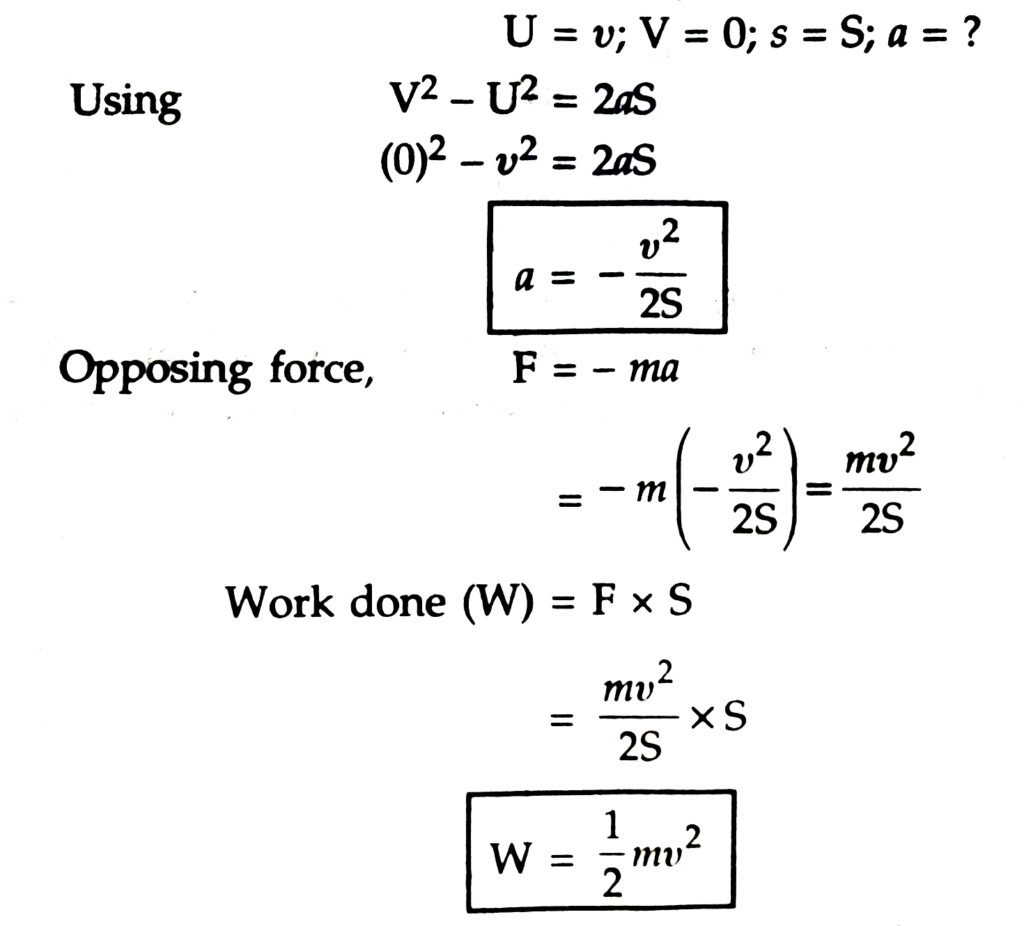
Q. 17. In each of the following force F is acting on an object of mass m. The direction of displacement is from west to east shown by the longer arrow. Observe the diagram carefully and state whether the work done by the force in negative, positive or zero
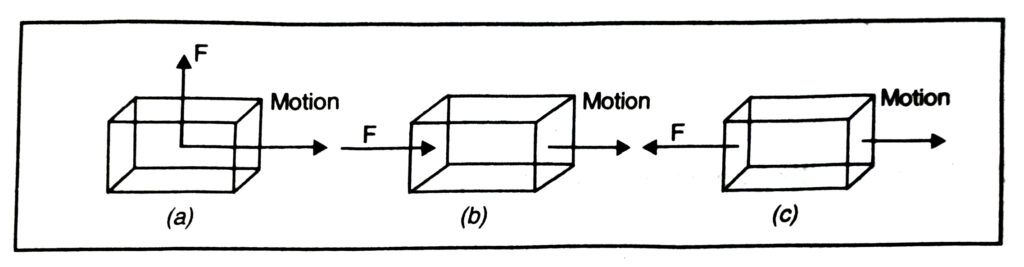
Ans.— (i) (a) displacement is in a direction perpendicular to direction of force applied, therefore work done is zero. There is no displacement in direction of force.
(ii) (b), the work done is positive since force and displacement are in the same direction.
(iii) (c), the work done is negative since displacement is in a direction opposite to direction of force.
Q. 18. Soni says that acceleration in an object could be zero even when several forces are acting on it. Do you agree with her ? Why ?
Ans.— Yes, acceleration can be zero when a large number of forces are acting on a body and their resultant is zero.
We know, a = F/m
= 0/m
∴ a = 0
Illustration. (i) If two equal and opposite forces are acting on an object, acceleration of the object is zero.
(ii) If three forces are simultaneously acting on an object and can be represented inmagnitude and direction by three sides of the triangle in the same order, the body is in equilibrium and will have zero acceleration even when 3 forces are acting on it.
(iii) Even with more than 3 forces acting on a body, it could have zero acceleration if the resultant of all force is zero.
Q. 19. A freely falling object eventually stops on reaching the ground. What happens to its kinetic energy ?
Ans.— A freely falling body on reaching the ground finally stops. Its kinetic energy gets transformed into other forms of energy such as heat, sound and light etc. and then into its potential energy.
ADDITIONAL IMPORTANT QUESTIONS
LONG ANSWER TYPE QUESTIONS
Q. 1. What is potential energy ? Deduce an expression for the gravitational potential energy of a body of mass ‘m’ placed at height ‘h’ above the ground. Give some practical examples.
Ans.— Potential Energy. It is the energy possessed by an object when it is raised above or below the surface of earth.
Mathematical Expression. Consider a body of mass ‘m’ raised to a height ‘h’ above the surface of earth to a position ‘B’. In order to lift the body to this height we have to apply minimum force equal to mg, the weight of the body in the upward direction from position ‘A’.

∴ Work done on the body against the
force of gravity (W) = Force × Displacement
W = F × S
But mg and S = h
W = mg x h
= mgh
The work done (W = mgh) on the body is
against the force of gravity
∴ Ep = W = mgh
Since this work done is against the force of gravity, so this energy is called gravitational potential energy. This potential energy does not depend on the path along which the body is moved.
Practical Examples. (i) In olden days there were no digital watches and the watches used to be wound up. When its spring is open then the watch does not work. When its spring is wound up then it starts working.
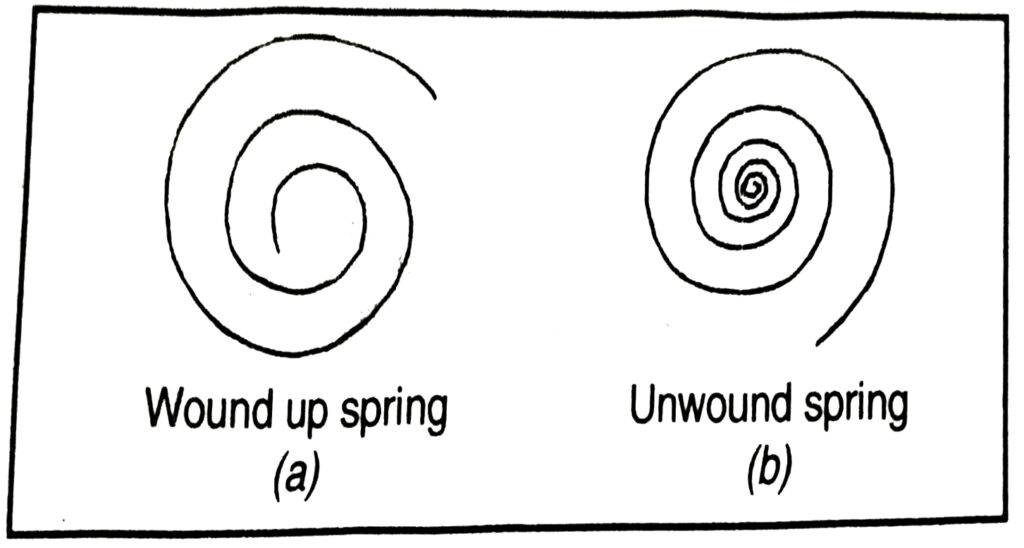
As is shown in Fig. on winding the spring closes and potential energy is stored up. This potential energy due to change in size and shape of the spring helps to move the hands of watch.
(ii) Try to open a spring with your both hands. Work is, therefore, being done by your hands. By doing this the spring extends in its length and as such potential energy is stored in it.
Q. 2. What is kinetic energy ? Derive a mathematical expression for kinetic energy.
Or
Define kinetic energy. Derive an expression for kinetic energy of an object of mass ‘m’ and moving with velocity ‘v’.
Or
Define kinetic energy and Potential Energy. Derive an expression for the kinetic energy of an object of mass ‘m’ moving with velocity ‘v’.
Ans.— Kinetic Energy. It is defined as the energy possessed by a body due to velocity. if body
is not in motion then it does not possess kinetic energy.
Potential Energy. Refer Q. No. 1 under Long Answer Type Quesitons on.
Mathematical Expression. Suppose a football of mass ‘m’ is at rest and force ‘F’ is applied. Due to the force acting on it, the football covers a distance ‘S’ in ‘t’ seconds and attains a velocity v. Thus, acceleration produced in the football is ‘a’
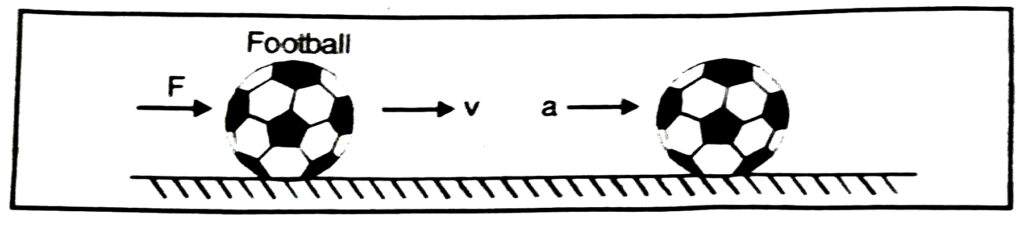
Work done on the football W = F × S
According to Newton’s second law of motion,
F = m × a

Equation (8) proves that the work done on the football is stored in it as kinetic enegry.∴
∴ Work done = kinetic energy stored = 1/2 mv²
Q. 3. What is law of conservation of energy ? Explain the law with the help of an example and prove its reality.
Ans.— Law of conservation of energy. According to this law total energy always remain constant or total energy always remains the same. Though one form of energy can be transformed to some other form of energy but total but total energy stil still remains constant.
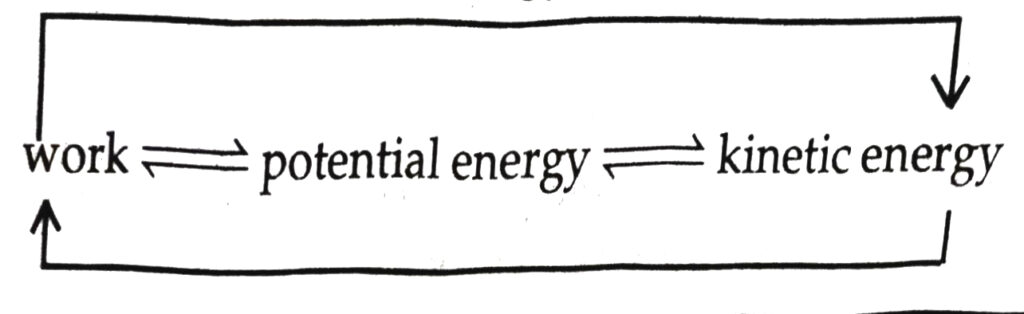
Explanation with example. Throw a ball vertically upwards from the surface of earth. You do some work on the ball. This work gets stored in the form of potential energy. This is called gravitational potential energy. As the ball moves up and up its velocity continue to decreaes and its kinetic energy continue to decrease and potential energy goes on increasing. When ball reaches the maximum height its kinetic energy becomes minimum i.e. zero but potential energy becomes maximum. We can prove mathematically that at any position in the path of motion of the ball. Sum of potential energy and kinetic energy remains same.

Proof for law of conservation of energy:
Consider a ball of mass m situated at maximum height above the earth surface. Let this ball be dropped from the point A as shown in fig. to reach the position C on the earth surface.
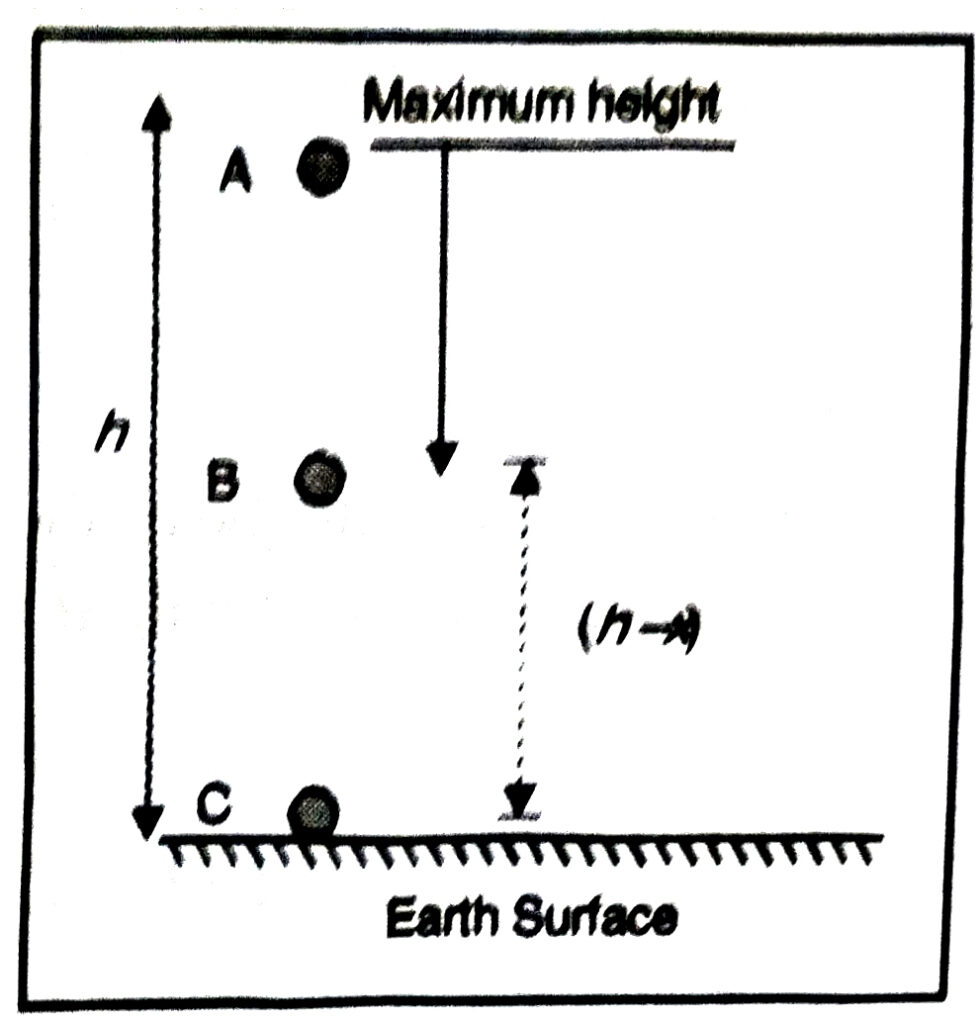
At point A

During the downward journey of the ball imagine that it is in position B and has travelled distance x. Let, v be the velocity of the ball when it reaches the position B.

At point B

Finally the ball strikes the earth surface at point C. Let v’ be the final velocity of ball at C.
At point C

Thus we find that each of positions A, B and C of the ball, total energy remains the same (= mgh) i.e., energy remains conserved.
On striking the earth surface the energy of the ball appears as sound and heat energy If by some means we are able to measure these forms of energy it would be found to be the same as at A or B or C. This proves the law of conservation of Energy.
Q. 4. If the force acting on the object is not in the direction of motion then how will you consider the work done ? Explain giving example and also tell when will the work done be minimum and when it will be maximum ?
Ans.— When the force acting on the object is not in the direction of motion. A gardener pushes the lawn mower in the forward direction. He applies force F on the handle HH’ facing the ground.

As is clear from the figure, the gardener is not applying force in the horizontal direction but applies force in a direction making angle θ with the direction of force. In such situation the force acting on the machine drives the roller in the horizontal direction from A to B.
Here the effective force is F cos θ and not F and the vertical component F sin θ balances the weight of the lawn mower.
∴ Work done by the lawn mower (W) = Component of Force × Displacement
= F cos θ × S
(i) When the force acts along the direction of displacement then θ = 0º and cos θ = cos 0º = 1
W = F cos θ × S
=F × 1 × S
W = F × S
This time the work done will be maximum.
(ii) When the force acting on the object is in a direction perpendicular to the direction of motion then θ = 90° and cos θ = cos 90º = θ W = F cos θ × S
∴ W = F cos θ 90º × S
= F cos 90° × S
= F × 0 × S
⇒ W = 0
This time the work done is minimum.
SHORT ANSWER TYPE QUESTIONS
Q. 1. What is work ? How can you calculate it ? Also give the unit of work.
Ans.— Work. Work done by a force or by an object in the product of applied force and the displacement taking place in the direction of applied force.
If F = force acting on the body
S = displacement of the body in the direction of force
work done by force, W = F × S ….(i)
we know, when a force acts on a body acceleration is produced in the body.
Thus, if m = mass of body
a = acceleration produced in the body then
from, Newton’s second law of motion
F = m × a ….(ii)
Using (i) and (ii),
W = m × a × S ….(iii)
Unit of work. When force is measured in Newton (N) and displacement in metre (m), the
Work = Newton × Metre
W = N × m
W = Joule (J)
∴ S.I. unit of work is Joule (J) and C.G.S. unit is erg.
1 Joule 107 erg.
Q. 2. Show by giving an example that if force acting in the body does not produce any displacement then the work done will be zero ?
Ans.— This statement can be understood by the following example. If a child tries to push a car by applying his, maximum force but the car does not displace even through 1 centimeter, then in the language of physics we can say that the child has done no work.
In this situation suppose the child applies force F and displacement S = 0 then
∴ Work done by the child, W = F × S
= F × 0
⇒ W = 0
Q. 3. A stone tied to one end of string is moved in a circle. How much work is done by the centripetal force in this circular motion ?
Ans.— A stone is made to move in a circle as shown the fig. then the finger with which you hold the string will experience some force. The force acting on the moving stone is known as centripetal force.
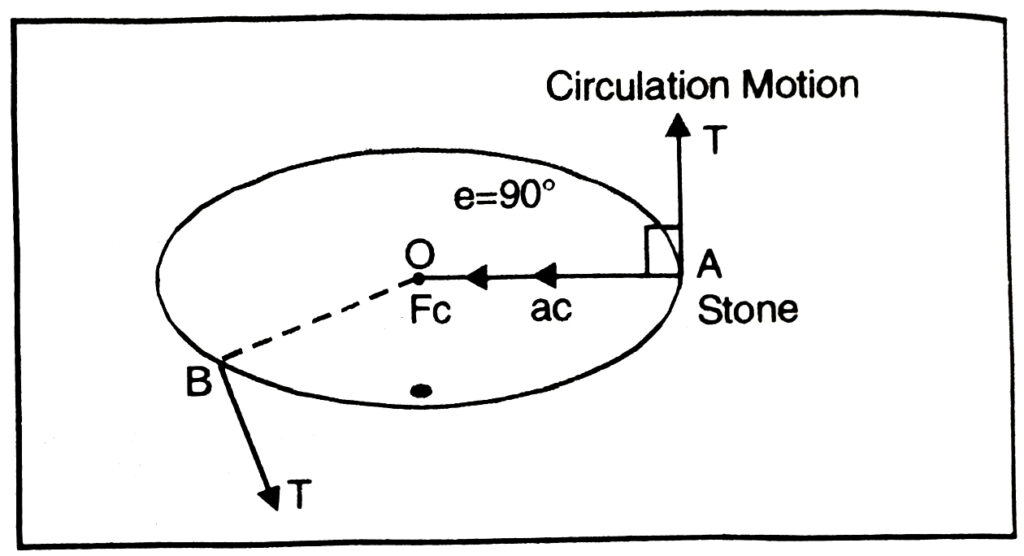
This force acts radially inwards towards the centre of the circle. If in this situation the stone gets detached then it will fly off in the direction tangential along AT or BT as shown in fig. 3.13 Now, the centripetal force acts perpendicular to the direction of motion, thus no work is done by the centripetal force.
Q. 4. What is power? Write its SI unit also.
Ans.— Power. Harish and Karan climbed up a tree to pluck 60 mangoes each. They started climbing at the same moment of time. Harish got his 60 mangoes in 30 minutes whereas Karan got his 60 mangoes in 60 minutes. That means Karan took more time to do the same work. Both did the same work, both had same energy but their power was 30. not same i.e. Power of Harish is more than that of Karan.
Power. Power of an object or a machine is its time rate of doing work i.e. Power is defined as rate of doing work with time.
∴ Power = Work done / time taken
or, P = W / t
If work is measured in joule and time in second then unit of power is ‘watt’.
1 watt = 1 Joule / 1 second
commercial unit of power is kilowatt.
1 kilowatt = 1000 watt = 1000 joule/second
Q. 5. Prove by an experiment that mechanical energy can be transformed into heat energy.
Or
When we hammer a nail into a wooden block the nail gets heated up? Why ?
Ans.—Place a nail on a wooden block and hammer it, some part of the nail goes into the wooden block, when nail is completely into the block due to hammering, when nail in further hammered then nail, hammer and block all gets heated up. When hammer is lifted up for hammering, its potential energy increases due to its position. When it falls on the nail then its whole energy is transformed into kinetic energy of the nail and the nail goes into the wooden block. When nail is completely embedded into the block then mechanical energy of the hammer converts into heat energy of the nail, block and hammer meaning thereby that mechanical energy gets converted or transformed into heat energy.
Q. 6. Differentiate between Potential Energy and Kinetic Energy.
Ans.— Difference between Potential energy (P.E.) and Kinetic energy (K.E.)
| Potential Energy | Kinetic Energy |
| The potential energy of an object depends upon its position and size. | The kinetic energy of an object is due to its motion or velocity. |
| Potential energy (P.E) = m × g × h | Kinetic energy (K.E.) = 1 / 2 mv² |
| Potential energy of an object depends upon its height above the ground or its depth below the ground surface. | The kinetic energy of an object depends upon its velocity. |
Q. 7. Define Kinetic energy and potential energy. Give one example of each.
Ans.— Kinetic energy. It is defined as the energy possessed by a body due to its velocity (motion).
Kinetic energy = 1/2 mv², where ‘m’ is the mass of the body and ‘v’ its velocity.
Example. 1. The bullet fired from the gun possesses kinetic energy due to its motion.
2. When an archer stretches the string of the bow then he does work which gets stood in the bow and arrow system as potential energy and when he releases the string, then this energy gets converted into kinetic energy of the arrow.
Potential energy. It is defined as the energy possessed by a body due to its raised position above the surface of earth or changed configurations.
Example. 1. The wound up spring of the watch possesses potential energy which when unwinds makes the various parts to the machinery to move.
2. A stone lying at the top of the rock possesses potential energy.
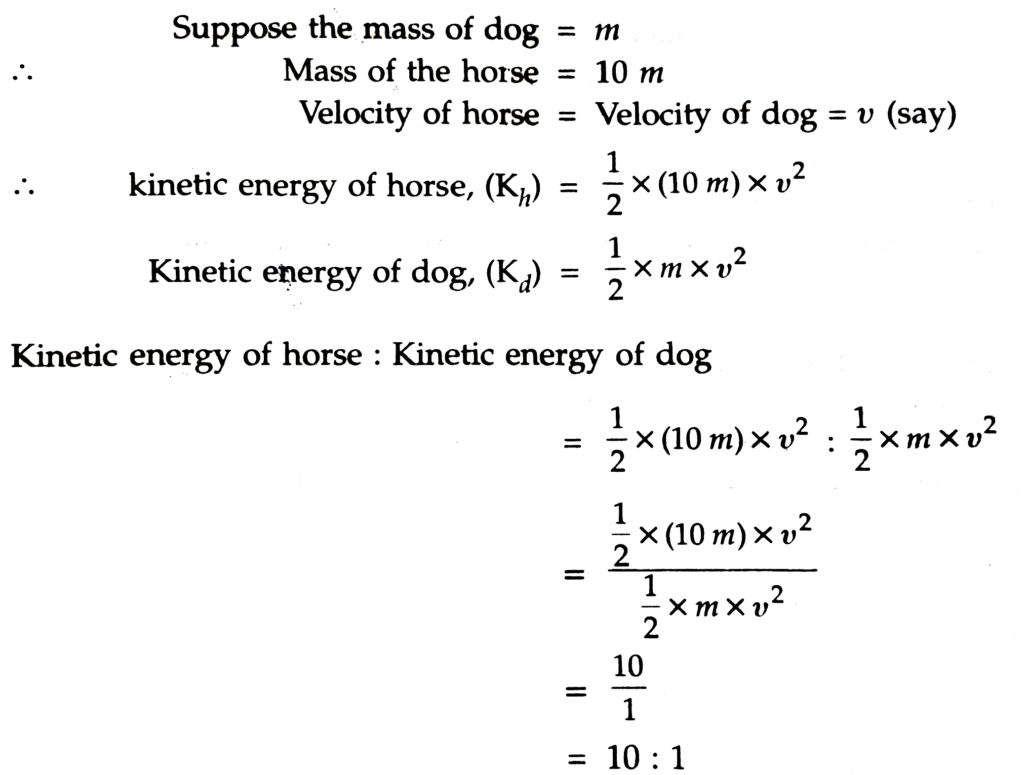
Q. 8. A horse and a dog are running with the same velocity. If the mass of horse is ten times the mass of the dog then what will be ratio of their kinetic energy ?
Sol.—
Q. 9. Two masses m and 2m are dropped from height h and 2h. On reaching the ground, which will have a greater kinetic energy and why ?
Ans.— K.E of mass m = P.E lost by mass m = mgh
K.E of mass 2m = P.E lost by mass 2m
= 2m × g × 2h = 4mgh
Hence, mass 2m will have a greater kinetic energy on reaching the ground.
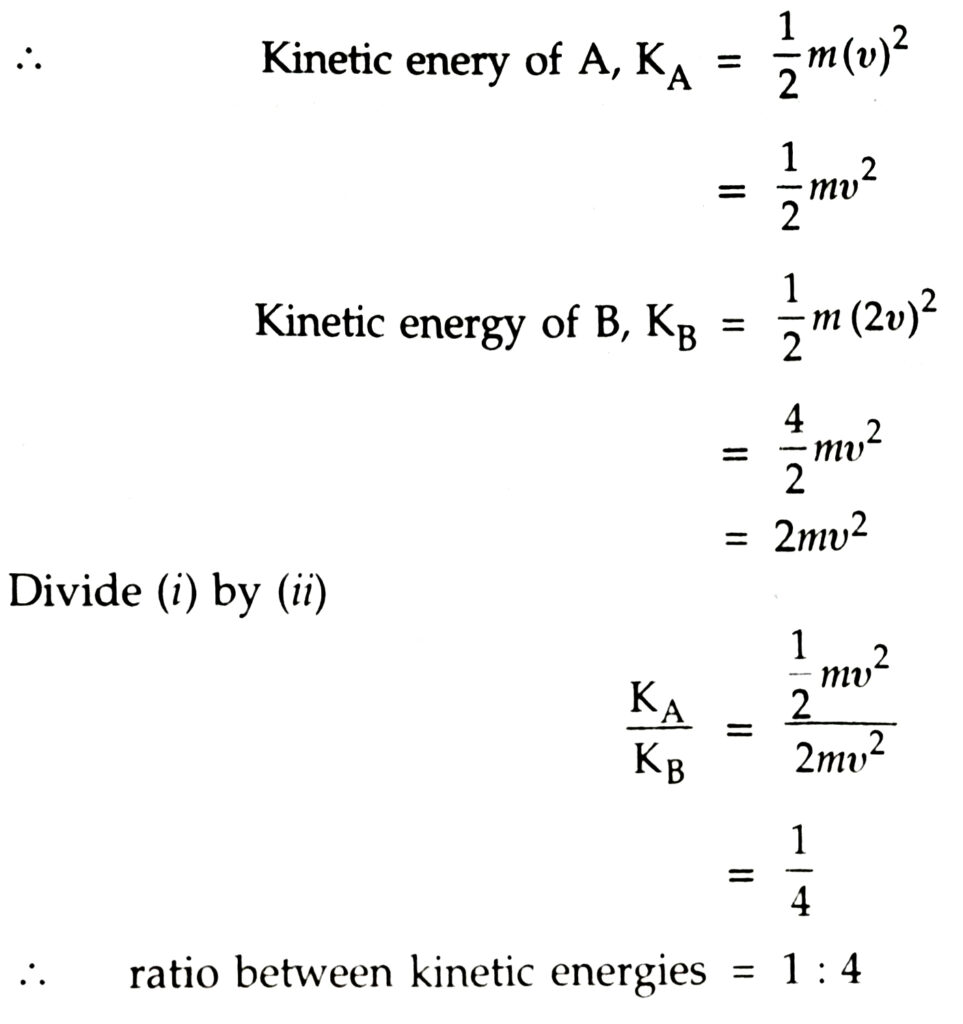
Q. 10. Two objects having same mass ‘m’ are moving with velocities v and 2v. Find ratio of their kinetic energies.
Ans.— Let bodies A and B each have same mass m and their respective velocities are v and 2v.
Q. 11. In what conditions the physical capacity of a man to do work decreases ?
Ans.— After sickness and in old age the physical capacity of a man to do work decrease because the energy of his body muscles becomes less.
Q. 12. Explain clearly the difference between energy and power with the help of an example.
Ans.— Energy. Energy is the ability to do work and is equal to the magnitude of total work which could be done by the energy. It has no relation with the time.
Power. It is the rate of doing work. It has no relation with the magnitude of total work.
Example. A worker taken one hour to complete a piece of work, whereas the other worker takes 2 hours to complete the same piece of work. In this case, both the workers are doing the same work i.e. both are consuming same amount of energy. But first worker did the same work in half the time as compared to other worker thus. First worker has doubled the power than the other worker.
NUMERICAL PROBLEMS (SOLVED)
Q. 1. 1 J of energy applied to lift a box of mass 0.5 kg. How much high it would be raised ?
Sol.— Potential energy (P.E) – 1 J
Mass of the box (m) = 0.5 kg
Acceleration due to gravity, (g) = 10 m/s2
Height to which box is raised, (h) = ?
We know, potential energy (P.E) = m × g × h
1 = 0.5 × 10 × h
1 = 5 × h
or, h = 1/5 = 0.2
∴ Height to which box would be raised, (h) = 0.2 m Ans.
Q. 2. A boy weighing 50 kg climbs up a vertical height of 100 m. Calculate the amount of work done by him. How much potential energy does he again ? Take r = 9.8 m/s2 ?
Sol.— Here, m = 50 kg, g = 9.8 m/s2 , h = 100 m
Work done by the boy = mgh
= 50 × 9.8 × 100
= 49000 J = 4.9 × 104 J
Gain in P.E = Work done = 4.9 × 104 J Ans.
Q. 3. A man drops a 10 kg rock from the top of a 20 m ladder. What will be its kinetic energy when it reaches the ground ? What will be its speed just before it hits the ground ? Does the speed depend on the mass of the rock ? (Take g = 10 ms2)
Sol.—
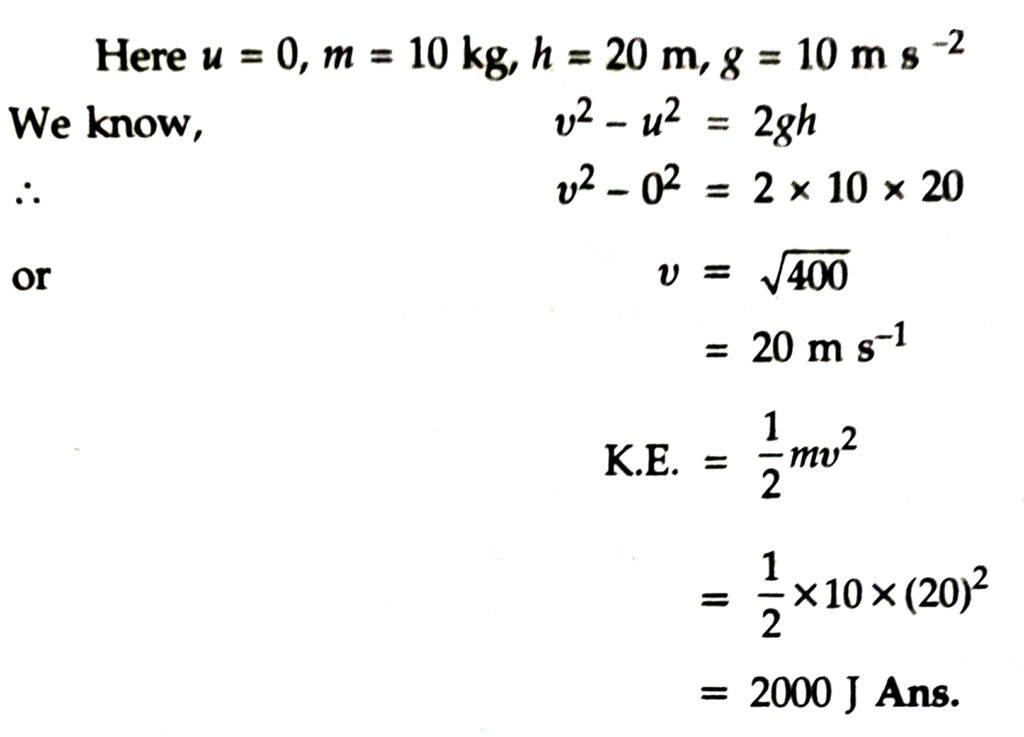
Speed does not depends on the mass of the rock because the acceleration due to gravity under which the rock falls does not depend on mass.
Q. 4. A rocket of 3 ×106 kg mass takes off from launching pad and acquires a vertical velocity of 1 km/s at an altitude of m25 km. Calculate the potential energy, and the kinetic energy. (Take g = 10 ms2)
Sol.—

Q. 5. An electric heater of 1000 W is used for 2 hours a day. What is the cost of using it for a month of 28 days, if 1 unit costs Rs. 3.00 ?
Sol.— Here, P = 1000 W
= 1 kW
Total time, t = 2 × 28 hours
= 56 hours
Total energy consumed = P × t
= 1 kW × 56 h
= 56 kWh
∴ Cost of 1 kWh = Rs. 3.00
∴ Cost of using electricity for Feb = 3 × 56
= Rs. 168. Ans.
Q. 6. The power of a motor pump is 5 kW. How much water per minute the pump an raise to height of 20 m ? (Take g = 10 ms2)
Sol.— Here, P = 5 kW = 5000 W, t = 1 min = 60s, h = 20 m, g = 10 ms-2
We know, P = W/t
= mgh / t
∴ Mass of water raised per minute, m = pt/gh
= 5000 × 60 / 10 × 20
= 1500 kg. Ans.
VERY SHORT ANSWER TYPE QUESTIONS
Q. 1. State the relation between commercial unit of energy and joules.
Ans.— 1 commercial unit of energy (or 1 kWh) = 3.6 × 106 Joule.
Q. 2. How much work is done on a body of mass 1 kg whirling on a circular path of radius 5 m ?
Ans.— Work done is zero.
Q. 3. What is the SI unit of power ?
Ans.— The SI unit of power is Watt.
Q. 4. A ball is thrown vertically upwards. Its velocity keeps on decreasing. What happens to its kinetic energy when it reaches the maximum height ?
Ans.— The kinetic energy of the body changes into its potential energy.
Q. 5. Define kilowatt hour.
Ans.— Kilowatt hour. It is defined as the work done or energy consumed when power of 1 kilowatt is consumed in 1 hour.
Q. 6. Name the term used for the sum of kinetic energy and potential energy of a body.
Ans.— Mechanical energy.
Q. 7. How many joules make one kilowatt hour ?
Ans.— 1 kilowatt hour = 3.6 × 106 J.
Q. 8. What should be the change in car city of a body required to increase its kinetic ? energy to four times of its initial value ?
Ans.— The velocity of the body should doubled at constant mass.
Q. 9. Under what conditions the work done by a force is zero inspite of displacement being taking place ?
Ans.— When displacement is in a direction perpendicular to the applied force.
Q. 10. What is the power of a machine which does 2000 joules of work in 10 seconds ?
Sol.— Power of a machine = Work done / time
= 2000 J / 10 s
= 200 Ans.
Q. 11. What is the SI unit of kinetic energy ?
Ans.— Joule.
Q. 12. Waterflows down the mountains to the plains. What happens to the potential energy of water ?
Ans.— Potential energy of water will decrease. It will change to kinetic energy of water.
MULTIPLE CHOICE QUESTIONS
Select the correct answer :
1. When mass is halved and velocity is doubled, the K.E. of a body :
(A) remains same
(B) is doubled
(C is 4 times
(D) is 1/4th.
Ans.— (B) is doubled
2. Kilowatt hour is the unit of :
(A) power
(B) force.
(C) electric energy
(D) pressure.
Ans.— (C) electric energy
3. Which one is not the unit of energy ?
(A) kilocalorie
(B) kWh
(C) erg
(D) watt.
Ans.— (D) watt.
4. When speed of a moving object is doubled, its :
(A) acceleration is doubled
(B) weight is doubled
(C) kinetic energy is doubled
(D) kinetic energy increases 4 times.
Ans.— (D) kinetic energy increases 4 times.
5. A body of mass 5 kg is rotated once around a circle of radius 4 m. Work done is :
(A) 20 J
(B) 40 J
(C) zero
(D) None of three is correct.
Ans.— (C) zero
6. SI unit of power is :
(A) J
(B) W
(C) N
(D) horse power.
Ans.— (B) W
7. Potential energy is :
(A) a scalar quantity
(B) a vector quantity
(C) neither scalar nor vector quantity
(D) scalar under one condition and vector under other state.
Ans.— (A) a scalar quantity
8. Two bodies of masses m₁ and m2 have equal kinetic energies. If P1and P2 are their respective momenta, then P1 : P2 is equal to :
Ans.— (A)
9. A spring is compressed. The potential energy of the compressed spring :
(A) remains unchanged
(B) increases
(C) increases
(D) becomes zero.
Ans.— (B) increases
10. The potential energy of a is maximum when she is :
(A) standing
(B) sitting on the ground
(C) sleeping on the ground
(D) siting in a chair.
Ans.— (A) standing
11. A stone is thrown vertically upward with a velocity v. The kinetic energy at the highest point is:
(A) 1/2 mv2
(B) zero
(C) 2{1/2 mv2}
(D) 2mgh.
Ans.— (B) zero
12. In a tug of war, work done by a winning team is :
(A) zero
(B) positive
(C) negative
(D) none of these.
Ans.— (B) positive
13. When a person retards t the motion of a body, the work done is :
(A) positive an
(B) zero
(C) negative
(D) none of these.
Ans.— (C) negative
Follow on Facebook page – Click Here
Google News join in – Click Here
Read More Asia News – Click Here
Read More Sports News – Click Here
Read More Crypto News – Click Here