PSEB Solutions for Class 10 Maths Chapter 12 Areas Related to Circles Ex 12.3

PSEB Solutions for Class 10 Maths Chapter 12 Areas Related to Circles Ex 12.3

PSEB 10th Class Maths Solutions Chapter 12 Areas Related to Circles Ex 12.3

Question 1.
Find the area of the shaded region in Fig., If PQ = 24 cm, PR =7 cm and O is the centre of the circle.

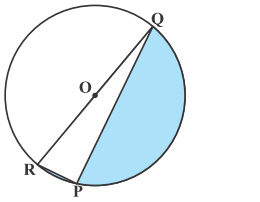
Question 2.
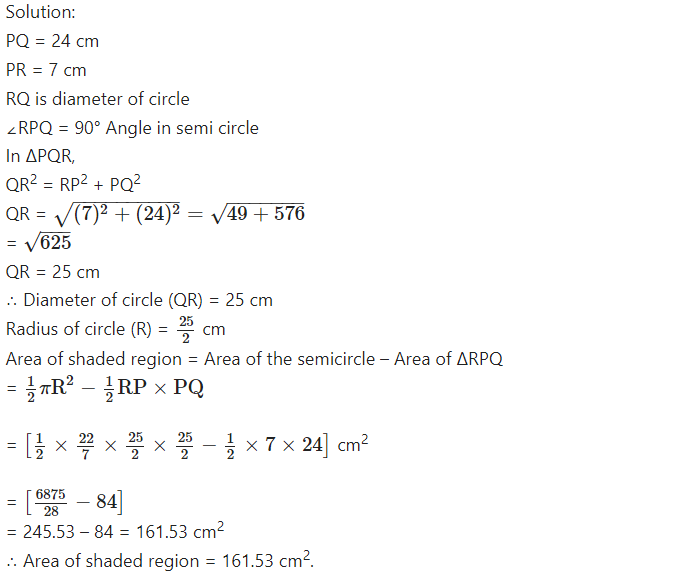
Find the area of the shaded region in Fig., if radii of the two concentric circles with centre O are 7 cm and 14 cm respectively and ∠AOC = 40°.

Question 3.
Find the area of the shaded region in fig., if ABCD is a square of side 14 cm and APD and BPC are semi circles.

Question 4.
Find the area of the shaded region in fig., where a circular arc of radius 6 cm has been drawn iith vertex O of an equilateral triangle OAB of side 12 cm as centre.

Shaded Area = Area of equilateral triangle OAB + Area of major sector of circle
= 62.28 + 94.28 = 62.28 cm²
Shaded Area = Area of equilateral triangle OAR + Area of major sectoç of circle
= 62.28 + 94.28 = 156.56
Shaded Area = 156.56 cm².

Question 5.
From each corner of a square of side 4 cm a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in fig. Find the area of the remaining portion of the square.
Solution:
Side of square = 4 cm
Radius of each semi circle cut out (r) = 1 cm

Question 6.
In a circular table cover of radius 32 cm, a design is formed leaving an equilateral triangle ABC in the middle as
shown in fig. Find the area of the design (shaded region).

Question 7.
In fig., ABCD is a square of side 14 cm. With centres A, B, C and D, four circles are drawn such that each circle touch externally two of the remaining three circles. Find the area of the shaded region.

Question 8.
Fig. depicts a racing track whose left and right ends are semicircular. The distahce between the two inner parallel line segments is 60 m and they are each 106 m long. 1f the track is 10 m wide, find
(i) the distance around the track along its inner edge.
(ii) the area of the track.

(i) Here AB = DC = 106 m
AF = BE = CG = HD = 10m
Diameter of inner semicircle (APD and BRC) =60 m
∴ Radius of inner semicircle (APD (r) = 30 m
Radius of outer semicircle (R) = r + 10 = 30 + 10 = 40 m
Distance around the track along inner edge = AB + circumference of semi circle BRC + CD + circumference of semi circle DPA
= 2 AB + 2 [circumference of semi circle BRC]
= 2 (106) + 2((2πr/2))
= 212 + 2πr
= 212 + 2 × 22/7 × 30
= 212 + 60×22/7
= 212 + 188.57 = 400.57 m.
∴ Distance around the track along its inner edge = 400.57 m

(ii) Area of track = Area of rectangle ABEF + Area of region BEMGCRB + Area of rectangle CGHD + area of region.
= 2 Area of rectangle ABCD + 2 Area of region (II)
= 2 (AB × AF) + 2
[Area of semi circle with Radius 60 cm – Area of semi circle with radius 30 cm]
= 2 [106 × 10] + 2 [latex]\frac{\pi \mathrm{R}^{2}}{2}-\frac{\pi r^{2}}{2}[/latex]
= 2 × 1060 + 2π/2 [R² – r²]
= 2120 + 22/7 (40² – 30²)
= 2120 + 22/7 [1600 – 900]
= 2120 + 22/7 [700]
= 2120 + 2200 = 4320 m²
Area of track = 4320 m²

Question 9.
In Fig., AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region.

Question 10.
The area of an equilateral triangle ABC is 17320.5 cm². With each vertex of the triangle as centre, a circle is drawn with radius equal to half of the length of the side of the triangle (see Fig.). Find the area of the shaded region.
(Use n = 3.14 and ,√3 = 1.73205)

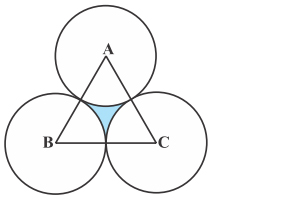
Solution:
Area of equilateral triangle ABC = 17320.5 cm²

Area of three sector = 3 × 15700/3 cm²
∴ Required shaded Area = Area of triangle – Area of three sectors
= 17320.5 – 15700 = 1620.5 cm²
∴ Hence, Required shaded Area = 1620.5 cm²

Question 11.
On a square handkerchief, nine circular designs each of radius 7 cm are made (see Fig). Find the area of the remaining portion of the handkerchief.

Question 12.
In Fig., OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the
(i) quadrant OACB,
(ii) shaded region.

Solution:

∴ Shaded Area = Area of quadrant OACB – Area of ∆ODB
= 9.625 – 3.5 = 6.125 cm²
∴ Hence, Shaded Area = 6.125 cm².

Question 13.
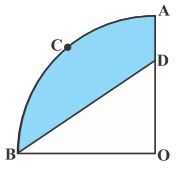
In fig., a square OABC is inscribed in a quadrant OPBQ. If OA = 20 cm, find the area of the shaded region.

Solution:
Side of square ABCO = 20 cm
∠AOC = 90°
AB = OA

Question 14.
AB and CD are respectively arcs of two concentric circles of radii 21 cm and 7 cm and centre O. If ZAOB = 30°, find the area of the shaded region.

Area of smaller sector (ODC) = 12.83 cm²
Now, Shaded Area = Area of bigger sector OAB – Area of smaller sector OCD
= 115.5 – 12.83 = 102.66
Hence, Shaded Area = 102.66 cm².

Question 15.
In fig., ABC is a quadrant of a circle of radius 14 cm and a semi circle is drawn with BC as diameter. Find the area of the shaded region.

Solution:
Radius of quadrant ACPB (r) = 14 cm
Sector angle (θ) = 90°
AB = AC = 14 cm

Required Area = Area of semi circle – [Area of sector – Area of ∆BAC]
= 154 – [154 – 98]
= (154 – 56) cm² = 98 cm²
Hence, Shaded Area = 98 cm².

Question 16.
Calculate the area of the designed region in fig. common between the two quadrants of circles of radius 8 cm each.

Area of sector = 50.28 cm²
Area of ∆ABD = 1/2 × AB × AD
= 1/2 × 8 × 8
= 32 cm²

∴ Area of segment DMBPD = Area of sector ∆BPD – Area of ∆ABD
= 50.28 – 32 = 18.28 cm²
Hence, Shaded area = 2 area of segment DMBPD = 2 (18.28) = 36.56 cm²

Follow on Facebook page – Click Here

Google News join in – Click Here

Read More Asia News – Click Here

Read More Sports News – Click Here

Read More Crypto News – Click Here