PSEB Solutions for Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.3

PSEB Solutions for Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.3

PSEB 10th Class Maths Solutions Chapter 8 Introduction to Trigonometry Ex 8.3

Question 1.
Evaluate:

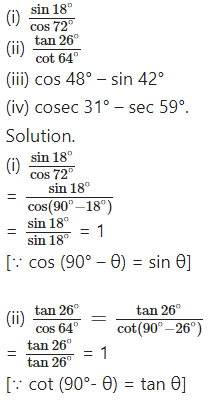
(iii) cos 48° – sin 42°
= cos (90° – 42°) – sin 42°
[∵ cos (90° – 0) = sin O]
= sin 42° – sin 42° = 0.

(iv) cosec 31° – sec 59°
=cosec 31° – sec (90° – 31°)
= cosec 31° – cosec 31°
[∵ sec (90° – θ) = cosec θ].

Question 2.
Show that:
(i) tan 4 tan 230 tan 42° tan 67° = 1
(ii) cos 38° cos 52° – sin 38° sin 52° = 0
Solution:

(ii) L.H.S.= cos 38° cos 52° – sin 38° sin 52°
= cos 38° × cos (90 – 38°) – sin 38° × sin (90° – 38°)
= cos 38° × sin 38° – sin 38° × cos 38
= 0.
∴ L.H.S. = RH.S.

Question 3.
If tan 2A = cot (A – 18°) where 2A is an acute angle, find the value of A.
Solution:
Given: tan 2A = cot (A – 18°)
⇒ cot (90° – 2A) = cot (A – 18°)
[cot (90° – θ) = tan θ]
⇒ 90°- 2A = A – 18°
⇒ 3A = 108°
⇒A = 36°.

Question 4.
If tan A = cot B, prove that A + B = 90°.
Solution:
Given that: tan A = cot B
⇒ tan A = tan(90° – B)
[∵ tan (90° – θ) = cot θ]
⇒ A = 90° – B.
⇒ A + B = 90°..

Question 5.
If sec 4A = cosec (A – 20°), where 4A is an acute angle, find the value of A.
Solution:
Given that: sec 4A = cosec (A – 20°)
⇒ cosec (90° – 4A) = cosec (A — 20°)
[∵ cosec (90° – θ) = sec θ]
⇒ 90° – 4A = A – 20°
⇒ 5A = 110°
⇒ A = 22°.

Question 7.
Express sin 67° + cos 75° in terms of Trigonometric ratios of angles between 0° and 45°.
Solution:
Given that: sin 67° + cos 75°
= sin (90° – 23°) + cos (90° – 15°)
= cos 23° + sin 15°
[∵ sin(90° – θ) = cos θ and cos (90° – θ) = sin θ].

Follow on Facebook page – Click Here

Google News join in – Click Here

Read More Asia News – Click Here

Read More Sports News – Click Here

Read More Crypto News – Click Here